Study a fractionally loaded heat equation with a load as a Riemann — Liouville derivative



We study the fractionally loaded heat equation with a load as a Riemann-Liouville derivative with respect to the time variable. The study of set boundary value problem is concluded with obtaining the integral equation. Also, we checked the limit cases for the fractional order derivative in the heat equation of BVP. It could be seen that the order of the fractional derivative is crucial in stating the existence and uniqueness of solutions.

Keywords :heat equation, fractional Riemann-Liouville derivative, Volterra integral equation.

1 Introduction

The differential theory and fractional integration have been developing thanks to their application in different fields of science. Noticeably, the fractional derivative study has been done actively in previous decades [1–4]. The interest in its study develops to grow too now [5–7]. Application of fractional derivatives, especially of Riemann-Liouville type, has been used in physics problems which involve impulses. The study of existence of higher order derivatives has been done in [5]. This paper is about a boundary value problem of a heat equation with the fractional load. The load is a Riemann-Liouville fractional derivative of the first order. Using the general solution obtained from [10] and manipulating through the solution the original problem has been reduced to a Volterra integral equation of a second kind with a kernel of degenerated hypergeometric Tricomi function. Later the limiting cases in the [0,1] interval it was proven that solution in the boundary values correspond to the kernel of the function.

2 Background definitions and concepts

It is better to revise some defined notions and concepts. Let us start from the definition of the fractional derivative of Riemann-Liouville type.

Definition 2.1 Let . Then, the Riemann-Liouville derivative of the order is defined as follows:

(1)

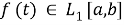
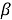
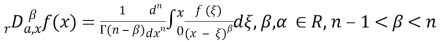
For and :

(2)

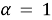
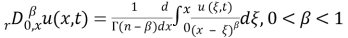
In addition, these following special functions are used during this paper:

is the integral of probabilities.

is the complementary integral of probabilities.

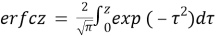
Definition 2.2 Linear independent solutions of the equation

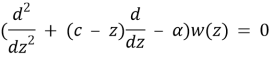
are functions , where is a degenerate hypergeometric function:

+

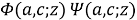
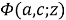
and is Tricomi degenerate hypergeometric function [8]:

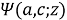
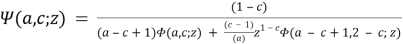
Tricomi degenerate hypergeometric function can be represented as an integral [9]:

(3)

For large values, an asymptotic formula holds:

where is a generalized hypergeometric series defined by the formula:

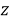
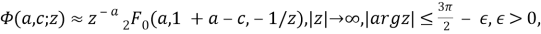
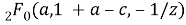
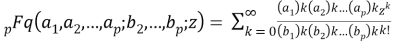
where

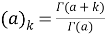
is the Pohammer symbol. Theory of differential equations with fractional derivatives has been a pivot point in the study of fractional calculus. Firstly, we will use the method of integral equations where the boundary values are brought down in two integral equations with transformed Kernel.

We also in this paper use a method to contemplate on our problem by reducing the differential part into integral equation. It was proven that in the domain the solution to the boundary value problem of the heat equation [10]:

,

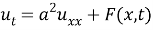
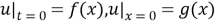
is described by the formula

,

where

(4)

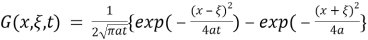
It will be seen further that the first and second terms in the equation (4) are equal to zero in correspondence with boundary values. So, then we will be left only with the third term, where is defined as:

(5)

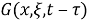
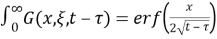
3 Statement of the problem

In the domain we have

,(6)

,(7)

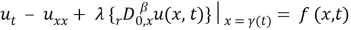
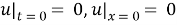
where is a complex parameter, is the Riemann-Liouville derivative in (2) of an order , is a continuous increasing function,

. The problem is carried in the class of continuous functions.

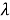
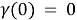
4 Reducing the boundary value problem to an integral equation

Lemma 4.1. The boundary value problem (6)-(7) is equivalently reduced to Volterra integral equation of the second kind with a kernel that contains a special function.

Proof. Let and . Applying boundary conditions in (7) to the solution of the heat equation with fractional load written in (4), we obtain:

(8)

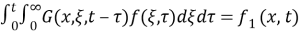
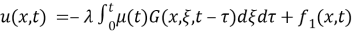
Then we take the fractional derivative of order Riemann-Liouville type with respect to two times. After that by applying the equality

, we obtain on the left side of the equation. Then, we will have the Riemann-Liouville derivative w.r.t. of order for the first term and for the second term, namely, we define .

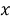
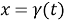
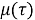
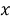
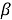
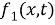
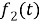
Thus (8) becomes as the following as we insert (5):

(9)

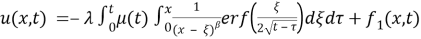
We calculate the derivative and by interchanging the integrals:

(10)

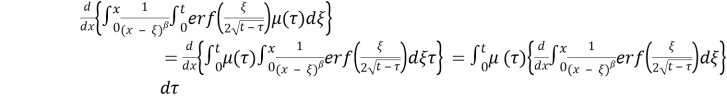
We introduce a new variable

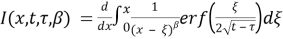
Then we rewrite the (10):

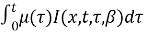
By change of the variable, we can solve :

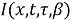
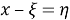
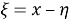
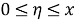
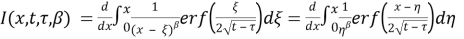
By taking the partial derivative:

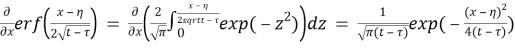
Hence, we will have:

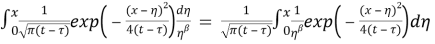
By substituting

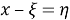
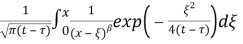
By using Gradstein’s solutions [8],

here is a degenerate hypergeometric sequence, where

.

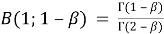
Then

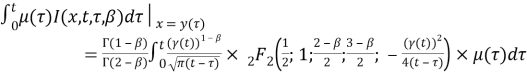
Then (8) becomes:

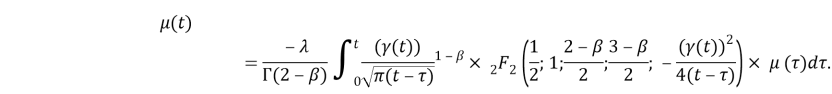
Then in such way BVP (6)-(7) is reduced to the following integral equation:

(11)

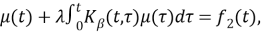
where

. (12)

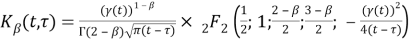
5 Study the boundary points of the changing interval of derivative order

Lemma 4.1. For boundary value problem (6)-(7) there is a continuity in the order of the derivative in the loaded term of equation (6).

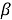
Proof. The boundary points of our problem (7) are considered. Now we check the boundary points of :

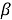
1. When , the problem is rewritten as

(13)

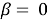
where

,

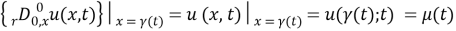
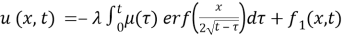
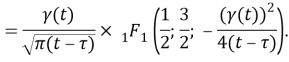
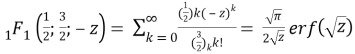
where

.

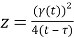
Hence,

.

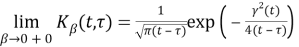
So,

II. Now, for a case :

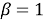
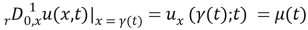
And then by taking the derivative w.r.t.

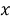
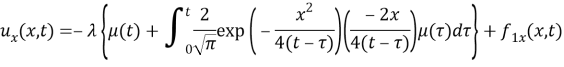
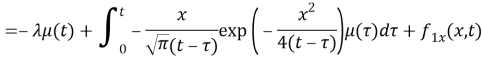
As we defined

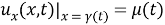
The equation becomes

(14)

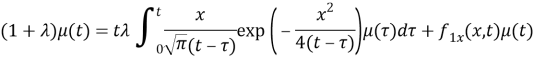
If we have the Volterra integral equation of the first kind, which can be reduced by differentiation to the Volterra integral equation of the second kind under certain conditions imposed on the kernel of the integral equation.

The Kernel of (14) has the form:

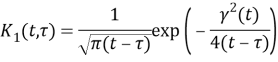
In another hand, by taking the limit from (12), we obtain:

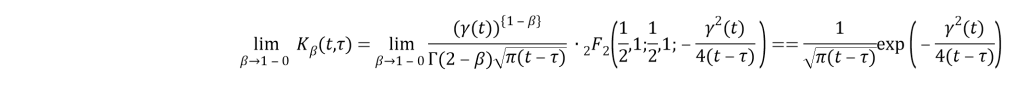
When , kernel (12) of the integral equation (11) is continuous for derivative order in its interval of changing.

6 Conclusion

Covered by theorems` s statement, kernel (12) of the integral equation has a weak singularity. Consequently, to find an unique solution to the equation (11) in the class of continuous functions, it is possible to apply method of successive approximations. Natural classes of functions are well-pose homogenous boundary value problems.

In case when ω ≥ 21 and ω ≥ 1 − 2β when 0 ≤ β ≤ 1 for at successive approximations cannot be used to solve integral equation (11). In several cases some values of the parameter may have different solutions from zero for the corresponding homogeneous equations. In case when uniqueness of solution to the first boundary value problem is violated the load can be identified as a strong perturbation, while in any other cases load is a weak perturbation.

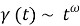
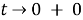
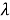
References:

1. Samko, S. G., Kilbas, A. A., & Marichev, O. I. (1993). Fractional Integrals and Derivatives. Theory and Application. Gordon and Breach: New York, 1006 p.

2. Le Mehaute, A., Tenreiro Machado, J. A., Trigeassou, J. C., & Sabatier, J. (2005). Fractional Differentiation and its Applications. Bordeaux Univ: Bordeaux.

3. Podlubny, I. (2002). Geometric and physical interpretation of fractional integration and fractional differentiation. Fract. Calculus Appl. Anal., 5, 367–386. an: 1042.26003.

4. Heymans, N., & Podlubny, I. (2006). Physical interpretation of initial conditions for fractional differential equations with Riemann-Liouville fractional derivatives. Rheologica Acta, 45 (5), 765–772. DOI: 10.1007/S00397–005–0043–5.

5. Feng, M., Zhang, X., & Ge, W. (2011). New existence results for higher-order nonlinear fractional differential equations with integral boundary conditions. Bound. Value Probl. Art., 720702, 20 pp. DOI: 10.1155/2011/720702.

6. Cao Labora, Daniel, Rodriguez-Lopez, Rosana, & Belmekki, Mohammed. (2020). Existence of solutions to nonlocal boundary value problems for fractional differential equations with impulses. Electronic Journal of Differential Equations, 15, 16 pp.

7. Yusuf, A., Qureshi, S., Inc, M., Aliyu, A.I., Baleanu, D., & Shaikh, A.A. (2018). Two-strain epidemic model involving fractional derivative with Mittag-Leffler kernel. Chaos: An Interdisciplinary Journal of Nonlinear Science, Vol. 28, Iss. 12, 28 pp. 123121. DOI: 10.1063/1.5074084.

8. Gradshteyn, I.S., & Ryzhik, I.M. (2007). Table of Integrals, Series, and Products. AP: New York. USA. 7 edition, 1171 p.

9. Prudnikov, A. P., Brychkov, Yu. A., & Marichev, O. I. (1989). Integrals and Series: More Special Functions. Vol. 3. Gordon and Breach: New York-London. 808 p

10. Polyanin, A.D. (2002). Handbook of Linear Partial Differential Equations for Engineers and Scientists. Chapman and Hall/CRC: New York-London. 780 p.