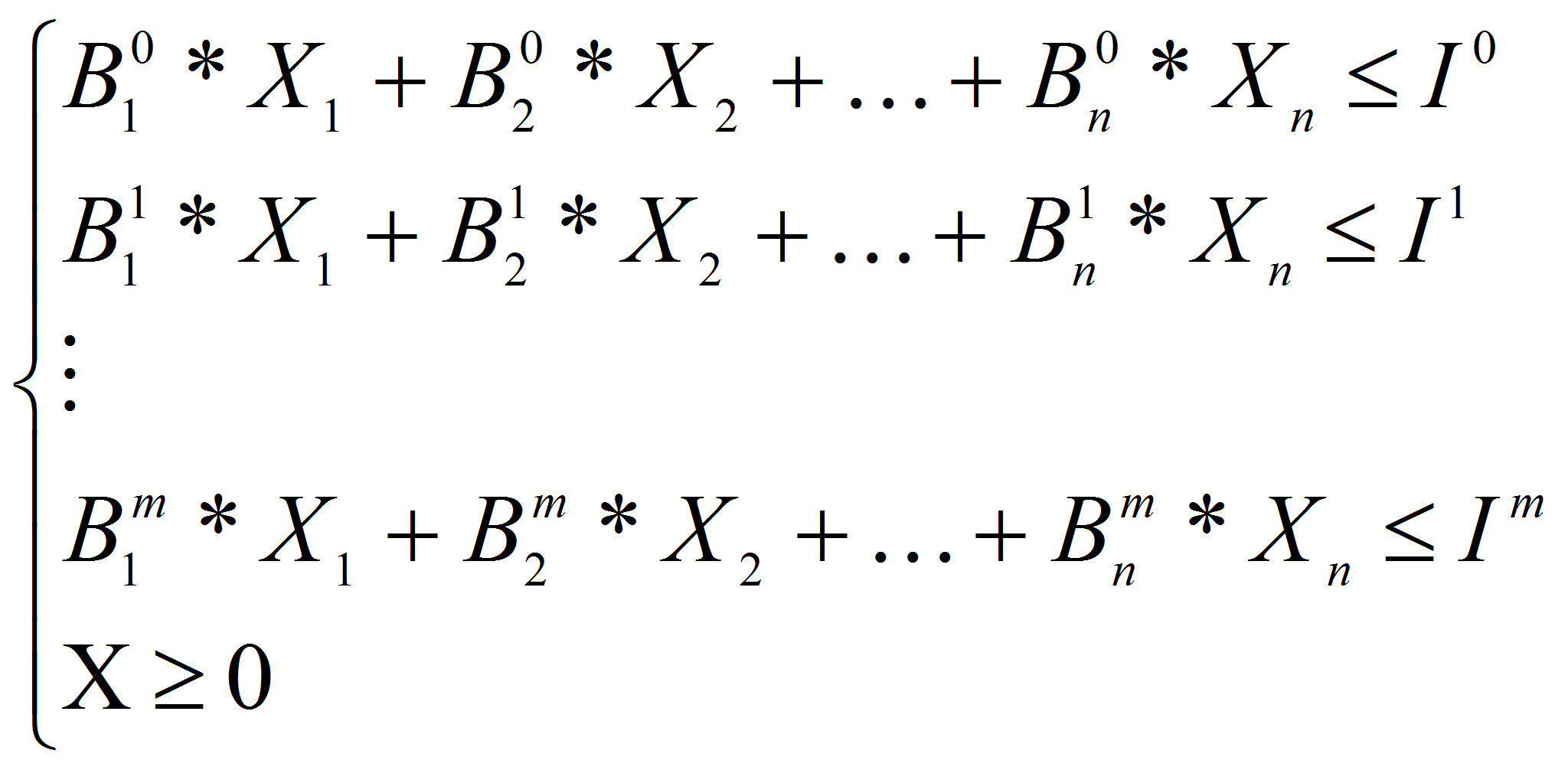The Analysis of Mathematical Model of Information System

The modern organization represents complex dynamic system. Perfection of information system is provided with use of modern means, the software and mathematical modeling.

Let's develop mathematical model of information system and we shall lead its analysis.

The mathematical model is formulated as follows: from among the firms, rendering services satellite Internet in territory of the Russian Federation, it is required to choose the provider satellite Internet with the maximal size of the net present value (NPV) and satisfying to financial restrictions.

The mathematical model includes two stages:

1. A method of expert estimations the firms giving satellite Internet in territory of the Russian Federation get out.

2. Methods of mathematical programming the optimum variant from among the satellite providers chosen at the first stage gets out.

Let - number of variants of projects satellite Internet; - number of the experts estimating variants of projects satellite Internet; -number of factors; - the weight appropriated q by the expert k to the factor, -an estimation given q by the expert k to the factor then the average estimation of i variant of the project satellite Internet is defined under the formula

(1)

At the second stage the optimum project from among the projects certain at the first stage gets out. The problem of linear programming of a choice of the optimum project from an investment portfolio is formulated.

The mathematical model of a choice of the optimum investment project satellite Internet looks like:

under restrictions

(2)

where is a target parameter, unit of measurement;

is investment expenses of i project in j period of time, million. roubles;

is available means of financing in j period of time, million. roubles;

is a share of financing of the investment project;

is a number of the investment project;

is a number of the period of time, year.

Let's carry out a choice of the optimum project satellite Internet for the District Compulsory Medical Insurance Fund of Kurgan region and we shall lead its analysis.

As a result of the first stage in a portfolio of investments are included by Joint-Stock Company " NTV-plus " (199 points), Europe Online Networks (EOL) (177 points), Astra Networks (157 points), Satpro (152 points) and Network Service (137 points).

Let's execute the second stage of mathematical model.

Let - a share of financing of the project NTV-plus, - a share of financing of project Europe On Line, - a share of financing of project Astra Network, - a share of financing of project Satpro, - a share of financing of project Network Service.

The mathematical model looks like

under restrictions (3)

The linear programming task is solved by Gauss – Jordan’s method [1, p. 107].

The optimum decision of a problem is resulted in table 1.

Let's lead the analysis of mathematical model.

Economic sense of additional variables - size of not used means of financing. Values of variables , , hence, are completely used means of financing during periods Values of variables and , hence, means of financing during periods are underused on 2,3881 and 1,5 million roubles.

The dual estimation of required variables shows presence/absence of financing i project. If the dual estimation is equal 0 financing of the project is made. If the dual estimation of variable is strictly positive, financing is not carried out. Dual estimations and have zero values, projects of NTV-plus and Astra Network are financed. Dual estimations , and are equal 0,2526, 1,8225 and 1,4259. Projects Europe On Line, Satpro and Network Service are not financed.

Table 1

The optimum decision of a problem

The dual estimation of variable defines deficiency of a resource. It shows on how many will increase NPV at increase j a resource for one unit. Dual estimations of variables and are equal 0,1768 and 0,2853. It means that at increase in the allocated means of financing at 1 million roubles during periods NPV will increase on 0,1768 and 0,2853 million roubles. During periods the increase in means of financing is not recommended (dual estimations of variables and have zero values).

The analysis of stability of the optimum decision is resulted in change of factors of criterion function in table 2.

The bottom border of an interval of the stability, equal -, corresponds to zero value of a required variable. If value of factor of criterion function changes within the limits of an interval of stability from bottom up to the top border value of criterion function changes. In case of an output abroad an interval of stability the size of criterion function and value of required variables varies [2, p. 20].

Table 2

Factors of criterion function of mathematical model

If variable accepts nonzero value in the optimum plan (, ) the factor at this variable (investment expenses i the project, , j the period of time ), changing within the limits of an interval of stability, will lead to change of criterion function, and distribution add financings it will be kept. If the size of factor at variable leaves for the top border of an interval of stability the share of financing j the project will increase due to shares of financing of other projects. Otherwise the share of financing j the project will be reduced, and shares of financing of other projects will increase.

If variable accepts zero value in case of change of factor of a variable inside of an interval of stability in the decision of a problem nothing will change. If the size of factor will leave for the top border of an interval of stability the given project is necessary to start for financing, reducing financing of other projects. For example, investment projects Europe On Line, Satpro and Network Service in the optimum decision have zero values of required variables (there is no financing). If NPV project Satpro will exceed 1,9676 million roubles the project will start to be financed. Similarly for projects Europe On Line and Network Service.

The analysis of stability of the optimum decision is resulted in change of the right parts of restrictions in the table. 3.

Table 3

Values of the right parts of system of restrictions

In the optimum plan of means of financing are underused in the periods of time and , because the top border of an interval of stability +. If available means of financing it is a lot of, change in an interval of stability does not change the list of financed projects, shares of financing and size NPV. At an output for the bottom border of an interval of stability shares of financing and size NPV vary the list of financed projects. For example, if means of financing of projects in the period of time will be less than 0,6119 million roubles the list of financed projects will change, shares of financing and size NPV. If means of financing are spent completely its change in an interval of stability does not change the list of financed projects, but changes a share of financing and size NPV. At an output abroad an interval of stability the list of financed projects, shares of financing and sizes NPV varies. For example, if means of financing in the period of time will change from 2,9305 million roubles up to 8,0740 million roubles financing of projects will not change (NTV Plus and Astra Network with values and ), but will change shares of financing and size NPV. If means of financing overstep the bounds of an interval the list of financed projects will change, share of financing and size NPV.

Results of the lead researches have allowed to draw following conclusions.

1. The mathematical model of optimization of the information systems is developed, allowing to reduce expenses and terms of designing of information systems and to raise validity of accepted decisions.

2. The analysis of variables and mathematical model on stability of factors of criterion function and values of the right parts of restrictions is lead.

References:

1. H. A. Taha. Operations Research: An Introduction. Seven Edition: Traslation From English, M.: Publishing House Williams, 2005. – 912 p.

2. Kochkina E.M., Radkovskaya E.V. Methods Of Research And Modelling Of National Economy. - Ekaterinburg, Publishing house Ural State Economic University, 2001. - 93 p.